Which of the Following Can Be Used to Prove That Triangle Efg Is Also a Right Triangle?
Surface area of Triangle
The area of a triangle is divers as the total space occupied by the three sides of a triangle in a two-dimensional plane. The bones formula for the area of a triangle is equal to half the product of its base of operations and height, i.e., A = i/2 × b × h. This formula is applicable to all types of triangles, whether information technology is a scalene triangle, an isosceles triangle, or an equilateral triangle. It should exist remembered that the base of operations and the height of a triangle are perpendicular to each other.
In this lesson, we will larn the surface area of triangle formulas for different types of triangles, forth with some examples.
| ane. | What is the Expanse of a Triangle? |
| 2. | Area of a Triangle Formula |
| three. | Area of Triangle Using Heron'southward Formula |
| iv. | Surface area of Triangle With 2 Sides and Included Angle |
| five. | How to Discover the Expanse of a Triangle? |
| 6. | FAQs on Surface area of Triangle |
What is the Area of a Triangle?
The area of a triangle is the region enclosed within the sides of the triangle. The surface area of a triangle varies from one triangle to some other depending on the length of the sides and the internal angles. The surface area of a triangle is expressed in foursquare units, like, mii, cmii, in2, and so on.
Surface area of a Triangle Formula
The area of a triangle can exist calculated using various formulas. For example, Heron'due south formula is used to calculate the triangle'due south expanse, when we know the length of all three sides. Trigonometric functions are besides used to find the area of a triangle when we know 2 sides and the angle formed between them. Still, the basic formula that is used to find the area of a triangle is:
Area of triangle = ane/2 × base × meridian
Discover the following figure to run into the base and height of a triangle.
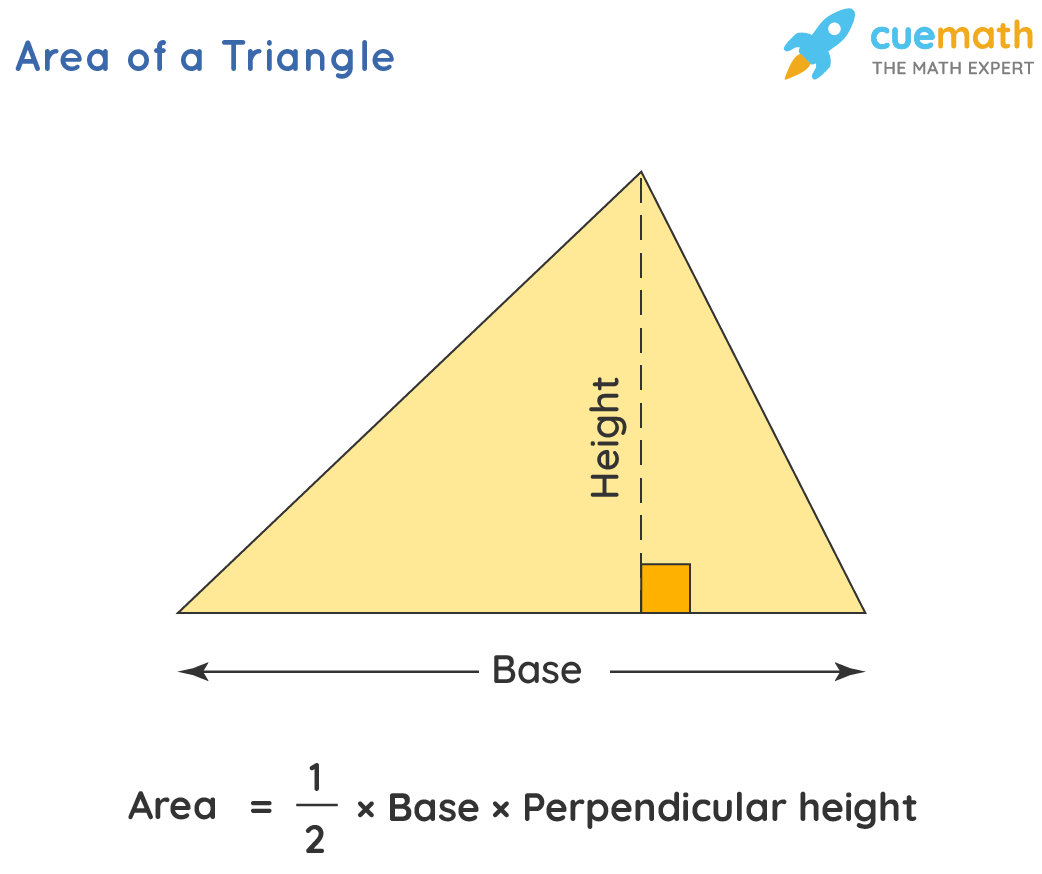
Let usa find the area of a triangle using this formula.
Example: What is the area of a triangle with base of operations 'b' = 2 cm and superlative 'h' = four cm?
Solution: Using the formula: Area of a Triangle, A = ane/2 × b × h = ane/two × 4 × 2 = iv cm2
Triangles can exist classified based on their angles as acute, obtuse, or right triangles. They can be scalene, isosceles, or equilateral triangles when classified based on their sides. Let us learn near the other ways that are used to notice the expanse of triangles with dissimilar scenarios and parameters.
Expanse of Triangle Using Heron'south Formula
Heron's formula is used to find the expanse of a triangle when the length of the iii sides of the triangle is known. To use this formula, nosotros need to know the perimeter of the triangle which is the distance covered around the triangle and is calculated past adding the length of all iii sides. Heron's formula has 2 important steps.
- Stride ane: Find the semi perimeter (half perimeter) of the given triangle by adding all three sides and dividing it past ii.
- Step two: Apply the value of the semi-perimeter of the triangle in the primary formula called 'Heron's Formula'.
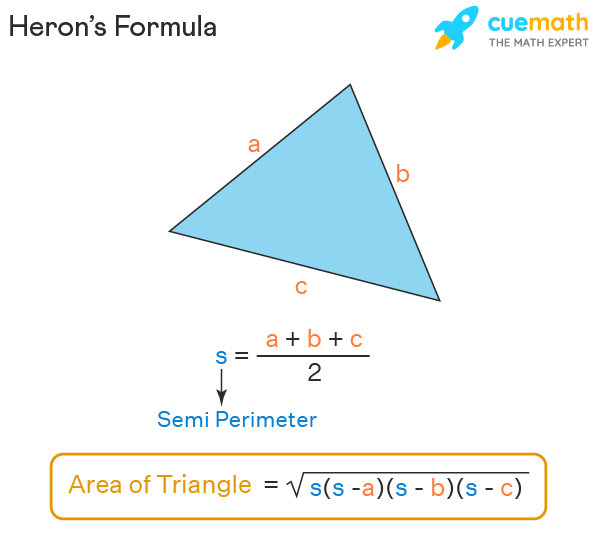
Consider the triangle ABC with side lengths a, b, and c. To detect the surface area of the triangle we use Heron's formula:
Expanse = \(\sqrt {s(south - a)(due south - b)(s - c)}\)
Notation that (a + b + c) is the perimeter of the triangle. Therefore, 'south' is the semi-perimeter which is: (a + b + c)/2
Area of Triangle With 2 Sides and Included Angle (SAS)
When two sides and the included angle of a triangle are given, we use a formula that has three variations co-ordinate to the given dimensions. For example, consider the triangle given below.

When sides 'b' and 'c' and included bending A is known, the area of the triangle is:
Expanse (∆ABC) = 1/2 × bc × sin(A)
When sides 'a' and 'b' and included angle C is known, the area of the triangle is:
Area (∆ABC) = 1/2 × ab × sin(C)
When sides 'a' and 'c' and included angle B is known, the surface area of the triangle is:
Expanse (∆ABC) = 1/two × air conditioning × sin(B)
Example: In ∆ABC, angle A = 30°, side 'b' = 4 units, side 'c' = 6 units.
Expanse (∆ABC) = 1/2 × bc × sin A
= 1/2 × 4 × 6 × sin 30º
= 12 × ane/2 (since sin 30º = 1/two)
Surface area = six square units.
How to Find the Area of a Triangle?
The area of a triangle can be calculated using various formulas depending upon the type of triangle and the given dimensions.
Expanse of Triangle Formulas
The area of triangle formulas for all the unlike types of triangles similar the equilateral triangle, right-angled triangle, and isosceles triangle are given below.
Area of a Correct-Angled Triangle
A right-angled triangle, also called a right triangle, has ane bending equal to 90° and the other ii acute angles sum up to 90°. Therefore, the height of the triangle is the length of the perpendicular side.
Expanse of a Right Triangle = A = 1/two × Base × Height
Area of an Equilateral Triangle
An equilateral triangle is a triangle where all the sides are equal. The perpendicular drawn from the vertex of the triangle to the base divides the base of operations into ii equal parts. To summate the area of the equilateral triangle, we need to know the measurement of its sides.
Area of an Equilateral Triangle = A = (√3)/four × side2
Surface area of an Isosceles Triangle
An isosceles triangle has 2 of its sides equal and the angles opposite the equal sides are also equal.
Expanse of an Isosceles Triangle = A = \(\frac{ane}{4}b\sqrt {4{a^2} - {b^2}}\)
where 'b' is the base and 'a' is the measure of ane of the equal sides.
Observe the table given beneath which summarizes all the formulas for the area of a triangle.
| Given Dimensions | Expanse of Triangle Formula |
|---|---|
| When the base and height of a triangle are given. | A = i/2 (base × height) |
| When the sides of a triangle are given as a, b, and c. | (Heron's formula) Surface area of a scalene triangle = \(\sqrt {s(s - a)(southward - b)(s - c)}\) where a, b, and c are the sides and 'southward' is the semi-perimeter; due south = (a + b + c)/ii |
| When two sides and the included angle is given. | A = one/2 × side 1 × side two × sin(θ) where θ is the angle between the given two sides |
| When base and height is given. | Area of a right-angled triangle = 1/2 × Base × Tiptop |
| When it is an equilateral triangle and one side is given. | Area of an equilateral triangle = (√three)/4 × side2 |
| When it is an isosceles triangle and an equal side and base is given. | Area of an isosceles triangle = 1/iv × b\(\sqrt {4{a^two} - {b^ii}}\) where 'b' is the base of operations and 'a' is the length of an equal side. |
Examples on Surface area of Triangle Formula
go to slidego to slidego to slide

Cracking learning in loftier schoolhouse using simple cues
Indulging in rote learning, you are likely to forget concepts. With Cuemath, you will acquire visually and exist surprised past the outcomes.
Volume a Free Trial Class
Practice Questions on Area of Triangle
become to slidego to slidego to slide
FAQs on Area Of Triangle
What is the Area of a Triangle?
The expanse of a triangle is the space enclosed past the iii sides of the triangle. It is calculated with the help of diverse formulas depending on the type of triangle and is expressed in square units like, cmii, inches2, and so on.
What is the Surface area of Triangle Formula?
The basic formula to find the surface area of a triangle is, area of triangle = i/2 (b × h); where 'b' is the base and 'h' is the meridian of the triangle. However, at that place are other formulas that are used to find the area of a triangle which depend upon the blazon of triangle and the known dimensions.
How to Observe the Area of a Triangle?
The area of a triangle can be calculated if the base and height of the triangle is given. The basic formula that is used to summate the area is, Area of triangle = 1/2 (base × height). In other scenarios, when other parameters are known, the post-obit formulas are used to find the area of a triangle:
- Area of a scalene triangle = \(\sqrt {due south(south - a)(southward - b)(south - c)}\); where a, b, and c are the sides and 's' is the semi-perimeter; southward = (a + b + c)/ii
- Expanse of triangle = i/2 × side ane × side 2 × sin(θ); when 2 sides and the included bending is known, where θ is the angle between the given two sides.
- Surface area of an equilateral triangle = (√3)/iv × side2
- Area of an isosceles triangle = 1/4 × b\(\sqrt {4{a^2} - {b^2}}\); where 'b' is the base and 'a' is the length of an equal side.
How to Discover the Base and Elevation of a Triangle?
The area of the triangle is calculated with the formula: A = 1/2 (base × height). Using the same formula, the height or the base can be calculated when the other dimensions are known. For example, if the expanse and the base of the triangle is known then the tiptop can exist calculated equally, Height of the triangle = (2 × Area)/base. Similarly, when the height and the area is known, the base of operations can be calculated with the formula, Base of the triangle = (two × Area)/height
How to Detect the Area and Perimeter of a Triangle?
The expanse of a triangle can be calculated with the help of the formula: A = one/2 (b × h). The perimeter of a triangle can be calculated by calculation the lengths of all the three sides of the triangle.
How to Find the Expanse of a Triangle Without Height?
The area of a triangle can exist calculated when only the length of the 3 sides of the triangle are known and the height is not given. In this instance, the Heron'due south formula can be used to detect the surface area of the triangle. Heron's formula: A = \(\sqrt {south(s - b)(s - b)(southward - c)}\) where a, b, and c are the sides of the triangle and 's' is the semi-perimeter; southward = (a + b + c)/two.
How to Find the Area of Triangle with Two Sides and an Included Angle?
In a triangle, when two sides and the included angle is given, and then the expanse of the triangle is one-half the product of the two sides and sine of the included bending. For example, In ∆ABC, when sides 'b' and 'c' and included bending A is known, the expanse of the triangle is calculated with the help of the formula: one/2 × b × c × sin(A). For a detailed explanation refer to the section, 'Expanse of Triangle With ii Sides and Included Angle (SAS)', given on this page.
How to Find the Area of a Triangle with 3 Sides?
The expanse of a triangle with 3 sides tin be calculated using Heron's formula. Heron's formula: A = \(\sqrt {southward(s - a)(s - b)(south - c)}\) where a, b, and c are the sides of the triangle and 'southward' is the semi-perimeter; s = (a + b + c)/2.
harndennottionged.blogspot.com
Source: https://www.cuemath.com/measurement/area-of-triangle/
0 Response to "Which of the Following Can Be Used to Prove That Triangle Efg Is Also a Right Triangle?"
Post a Comment